The Hydrino Hypothesis: Chapter 3

An Introduction To The Grand Unified Theory Of Classical Physics

This monograph is an introduction to Randell L. Mills’ Grand Unified Theory of Classical Physics, Hydrino science, and the efforts of the company Brilliant Light Power (BLP) to commercialize Hydrino-based power technology, as told by Professor Jonathan Phillips. Out of necessity, it assumes a degree of familiarity with physics and physics history. An overview of the BLP story which serves as a helpful introductory piece to those unfamiliar with its sweeping scope can be found here. Readers should also read the previous chapters of this monograph prior to this one:

Chapter 1 of The Hydrino Hypothesis

Chapter 2 of The Hydrino Hypothesis

By Professor Jonathan Phillips

An Introduction To SQM & The GUTCP

Preface- The purpose of this chapter is to provide a simple non-mathematical explanation of the fundamentals of the GUTCP in order to help re-frame the discussion and overcome the misinformation that pervades mainstream physics regarding both SQM and the GUTCP.

The Grand Unified Theory of Classical Physics (GUTCP), the new atomic theory upon which Brilliant Light Power’s (BLP) Hydrino technology is founded, and Standard Quantum Mechanics (SQM), the universally taught and employed quantum model, are based on two completely different paradigms. 

In the GUTCP all facets of the behavior of atoms and ions can be explained using equations developed before 1872 (classical physics), and all particles in the universe are three-dimensional objects of precise shape.  In the GUTCP model, classical physics pertains at all length and time scales. No deviation from these validated rules is permitted in GUTCP models, hence it is a true First Principles model.

In contrast to the GUTCP, SQM, as generally understood, throws out classical physics for its description of atoms, molecules, etc. and postulates the particles of the quantum world are not standard physical objects.  According to SQM, a non-classical equation, the Schrodinger Equation, first proposed in 1925, is used to describe the proposed non-physical nature of elementary particles. The solutions to the Schrodinger equation for hydrogen are probability distributions for infinitely small electrons that do not obey classical physics laws.

The probabilistic nature of quantum mechanics, where outcomes are predicted in terms of probabilities rather than certainties, was philosophically unsettling to many scientists at the time of its introduction.

In the context of this philosophical and scientific maelstrom, the idea of "hidden variables" was proposed. The central tenet behind hidden variables is that the apparent randomness and unpredictability in quantum mechanics arises due to our ignorance of some underlying yet undiscovered deterministic variables. If these "hidden" variables were known, the argument goes, the outcomes of quantum experiments would be as predictable as those in classical mechanics.

The GUTCP postulate that elementary particles are extended objects of precise shape is possibly the hidden variable concept: someday quantum and standard physics are united by the same laws, so long sought.  That is, the true hidden variable is a complete paradigm shift based on properly describing the electron, as well as other elementary particles, as extended physical objects of precise shape that obey classical physics, that is, the GUTCP!

Spectroscopy

In order to explain the need for quantum mechanics, it is necessary to introduce the tool of spectroscopy because almost all knowledge regarding the quantization of energy at the atomic level originates with spectroscopic data.

It is through the analysis of the light emerging when electrons in “excited” (above ground state energy) atomic states fall to a lower energy state or into the very lowest known energy level (the “ground state”), or light emitted when electrons are recaptured by ionized atoms, that we learn there are discrete allowed energies for electrons in atoms. 

If there were no quantization of energy, then the light emerging would be continuous and all wavelengths would be observed. In fact, spectroscopy shows that light is emitted only at very specific energies.

Excited States

What is an excited state?  An excited state occurs when an electron temporarily occupies an energy state greater than its ground state. All excited states of atoms are unstable, and only exist for a small fraction of a second,  whereas the ground states can be stable “forever.” An electron can become excited if it is given extra energy, such as if it absorbs a packet of light (a photon).  Figure 3-1 is a stylized visualization of what happens when an electron jumps to an excited state:

Figure 3-1: Electron excitation in an atom.  Representation (per the Bohr model) of an excited state after a ground state electron absorbs a photon. Note: the farther an electron is from the nucleus, the higher its energy level.

An electron falling back to the ground state releases a photon with the same energy as that which was absorbed during the excitation process (Figure 3-2):

Figure 3-2: Electron returning to ground state in an atom. Representation of an excited state electron falling to the ground state and releasing a photon.

Hydrogen Energy Levels

Consider how spectroscopy is employed to determine the excited state energy levels in hydrogen. 

In a simple case, pure molecular hydrogen gas is placed in a partially transparent chamber between electrodes.  Once voltage is applied to the electrodes, the molecular hydrogen, in part, breaks down into atoms, and in turn the atoms into ions (H⁺) and electrons (e^-).  Neither charged species is long-lived, as the hydrogen ions quickly re-capture electrons and in the process release photons.

This process repeats rapidly such that there is only a small steady state concentration of ions.  For example, at any moment in a low pressure microwave-generated discharge, no more than 1 in 10,000 hydrogen atoms are in an ionic state.¹²

The photons released as excited electrons fall to lower energy states or are re-captured can be analyzed. The spectrum analysis is based on the observed angle of diffraction; however, it is well established that the angle of diffraction is a function of the wavelength.  The shorter the wavelength, the higher the diffraction angle, and the higher the energy of the light.

The energy of the visible light spectrum increases according to the acronym ROYGBIV, with R, red, having the longest wavelength and the lowest energy and V, violet, the shortest wavelength and highest energy.   

In some cases of spectrum analysis, the angle of diffraction is reported, in others, only the energy of the observed quantized diffractions are reported.  They are all related by simple algebra, so the report dimensions of angle, wavelength, and energy are only selected as a matter of convenience. 

The reader may understand better by examining a typical report for atomic hydrogen seen in Figure 3-3 below. In this case wavelength was the dimension selected for the report (ordinate). 

The figure below shows the wavelengths in the visible spectrum observed when hydrogen electrons fall from a higher energy state to a lower energy state is not continuous.  The energy corresponds to quantized angles/wavelengths/energies/colors. 

That is, the energy release by hydrogen electron excitation and the subsequent fall back into a lower energy state is clearly quantized in the form of photons at very specific wavelengths. Those spectral lines form a pattern that is observed for hydrogen under all circumstances.  This pattern of angles/wavelengths/energies/colors is a fingerprint of hydrogen, distinct from all other atoms and molecules. 

In particular, the quantized energy levels (some energetically higher than the range of human vision, e.g. Lyman lines) found are the basis of the Rydberg formula, which will be discussed later.   

Figure 3-3: Atomic hydrogen Balmer series lines from a microwave plasma.

The spectroscopic lines in Figure 3-3 observed from atomic hydrogen generated in a microwave plasma are labelled 3-2, 4-2, 5-2 and 6-2.

Each designation represents the light emitted when electrons in allowed quantum excited state of atomic hydrogen (n=3,4,5,6, where n is the principle quantum number) naturally drop down to a lower energy level (n=2). The energy drop from higher allowed states to the next-to-lowest allowed state (n=2) is called the Balmer series. The other lines (OH) are “noise” arising from water in the chamber.

The higher the energy of the excited initial state, with “6” being the highest initial energy level shown, the shorter the wavelength and the higher the energy of the emitted photon as the electron returns to a lower energy level.

Each panel in Figure 3-3 shows a different relative intensity because of Hydrino-related effects predicted by the GUTCP for the plasma conditions associated with panels (a) through (e). Only panels (f) and (g) show the relative intensity associated with a normal thermodynamically equilibrated population of energy states (more on this later).

Spectrum Analysis

Precisely how is a spectrum analysis performed?  How is a mix of colors arising from a hydrogen plasma broken down into component wavelengths/energies?  An intuitive starting point is to consider the glass prism that takes ordinary sunlight and breaks it up into many colors.

Virtually everyone has witnessed a prism decomposing sunlight into an array of colors and thus should understand the concept that most light sources (exceptions include lasers) are actually composed of many distinct colors/energy photons. The prism acts to make the constituent photons of different wavelengths in sunlight bend at a different angle, hence allowing the observer to discover sunlight actually is composed of photons of many energy levels/colors. 

Another classic example are water drops in the atmosphere breaking down sunlight into an array of colors, i.e. a rainbow.

A quality visible light spectrometer uses not a prism, nor raindrops, but rather a very precisely generated tool called a diffraction grating. It consists of regularly spaced reflective surfaces, on a micrometer scale, for a reflecting grating, or transparent slits for a transmission grating. 

As shown in Figure 3-3, all light frequencies/colors in the light generated from hydrogen containing plasmas, after passage through, or reflections, from a high quality grating, are found at particular angles, corresponding to particular energies-colors. One clear feature of Figure 3-3 is that most of the lines arise from hydrogen atoms.

After many years of scientific study, the characteristic spectra of virtually all atoms, and many molecules are well known.

And clearly, not all these hydrogen containing plasmas are the same.  That is, all show the same colors, but the relative intensities are distinct.  All differences in the light pattern found from plasmas can be interpreted to provide insight into the chemistry and physics of the plasmas.

Of particular importance in this monograph will be the consistency or non-consistency of spectral features, particularly those arising from hydrogen, with SQM or GUTCP-based predictions.  

In Figure 3-3, in all panels the same wavelengths/energy levels for the Balmer series lines for hydrogen, generated by transitions from higher energy excited states (n=3,4,5,6…) to the penultimate lower energy hydrogen quantum state (n=2) are found (Balmer series).

However, only panels (f) and (g), plasmas containing no Hydrino catalyst species, have the normal relative intensities for the Balmer lines.  Specifically, in panels (f) and (g) the higher the energy/the greater the n-value, the smaller the population/shorter the line.  In contrast, for panels (a) through (e), all from plasmas containing a Hydrino catalyst species, there is a clear non-equilibrium population of excited state.  For example, in Panel (a) the level n=5 is more populated than the n=3 level. 

Why is the non-equilibrium population of excited states from plasmas containing a Hydrino catalyst significant? In Chapter 8 of this monograph the non-thermal equilibrium Balmer series intensity pattern (i.e. panels (a) through (e)) is shown to be inconsistent with SQM, but actually predicted by the GUTCP.  Later in this monograph this will be shown to be only one of many experimental results only consistent with the GUTCP prediction of Hydrino formation.

Figure 3-4: Compound H-alpha Balmer Lines from GEC RF Plasma. 

Figure 3-4 shows the H-alpha line (i.e. n=2 line in Figure 3-3) of the Balmer series.  This line was separated out from all the other plasma light with a very high quality diffraction grating, so high quality that even the shape of the line was captured.  A high-quality diffractometer using multiple diffraction gratings (Figure 3-5) provides a very precise measure of the frequencies created during electron capture.  For example the H-alpha line of the Balmer series can be shown to be at 656.3 +/-.005 nm, as seen in Figure 3-4. 

Of particular significance for later discussions of Hydrino formation is the clear evidence the spectral line in Figure 3-4 is “compound,” with a “thin” part from standard H atoms (~30%), and a very broad component (~70%) arising from superhot (meaning very fast-moving) hydrogen atoms.  As discussed later in this monograph, the existence of superhot hydrogen is another predicted outcome of the GUTCP, one impossible to explain using SQM. 

In sum, above (Figure 3-3 and 3-4) are demonstrated these features of atomic spectra:

• Angles/wavelengths/quantized energy levels that comprise a distinct fingerprint for each atomic species.

• Relative line intensities.

• Line shapes.

All quantum models are primarily focused on the first aspect, explaining the quantized energy levels revealed by spectroscopy. 

In Chapter 4 of this monograph it is demonstrated that the current leading quantum paradigm, SQM, and its many “approximate” versions, despite employing two or more variable parameters (i.e. curve fitting), do a very poor job of explaining the quantized energy levels revealed with spectroscopy for atoms with two or more electrons. 

In contrast, it will be shown in Chapters 4, 5, and 6 of this monograph that the GUTCP does an excellent job of predicting the quantized energy levels of every atomic species revealed with spectroscopy.  The GUTCP requires no variable parameters and is based on classic physics equations. In fact, for atomic species all spectroscopy can be determined using a few algebraic equations; no powerful computer required.   

Figure 3-5: Stylized illustration of two types of diffraction gratings.

The other features of spectra, particularly for hydrogen, will be shown to only be consistent with Hydrino formation.  For example, in Chapter 8 of this monograph, a lengthy explanation for the observed symmetric line broadening of atomic hydrogen, such as that shown in Figure 3-4, will be shown to be predicted by the GUTCP, but requires very “complex” explanations from standard physics.  Also, the non-standard relative intensity of hydrogen lines, per Figure 3-4, can be easily explained with the GUTCP, but not with SQM.

There is much to consider regarding diffraction gratings and their integration into a proper and precise spectrometer.  For this reason, the reader is referred to the literature on the topic.  Most books on diffraction are focused on very short wavelength x-ray spectrometers as those are employed to study the structure of crystals based on the diffraction spectra they produce. Still, the interested reader can garner more detail about the fundamental physics of diffraction, including for visible light, from the literature on the topic. 

SQM Interpretation Of Atomic Hydrogen Spectra

Like all the early quantum models, SQM was designed to explain the Rydberg empirical mathematical description of the energy level of the hydrogen atom. The series does not directly produce the observed spectra, but rather the allowed energy levels that can be teased out of the spectra.  The Rydberg formula is as follows:

Where R = 109,677 cm^-1, n_f = 1,2,3,…, n_i = 2,3,4,…, and n_i > n_f.

Bohr, Schrodinger, and Heisenberg each developed a theory for atomic hydrogen that gave the energy levels in agreement with Rydberg’s equation:

It must be pointed out that the Rydberg series represents a kind of “numerology.” That is, the series was not discovered on the basis of some theory of physics, but rather devised to fit a set of energies derived from experimentally observed spectral lines. 

A simple algebraic description of quantized energy levels is unique to hydrogen.  There is no similar algebraic model for quantized energy levels for any other atom.  For example, there is no algebraic model to describe the energy levels in helium as discussed in Chapter 4.

The basic Schrodinger model explains some aspects of the atomic hydrogen spectra quantitatively, and this is what provided the initial demonstration of its viability. Yet the caveats to SQM, as noted above, for even describing the hydrogen spectra accurately are serious. 

Indeed, SQM does not explain many aspects of one electron systems.  For example, it does not explain observed magnetic behavior. Also, as discussed in later chapters regarding the Hydrino hypothesis, none of the models can even explain the stability of the electron in the so-called ground state of atomic hydrogen (-13.6 eV). Moreover, to fit excited state energies of hydrogen (and only hydrogen) the proper relativistic form of the Schrodinger equation, the so-called Dirac equation is preferred.  A final general limitation of SQM and its many approximations: none, not the original Schrodinger equation nor any of its derivative approximations, can quantitatively predict any aspect of the spectra of any atomic species with two or more electrons, not even helium. The real Schrodinger equation is not even “solvable” once two or more electrons are present in the system.

So, how is SQM applied to multi-electron systems to explain the energy levels observed?  It is by curve fitting.  That is, the models always include two or more parameters that are adjusted until the energies predicted are similar to those observed. 

In sum, SQM was born to describe the hydrogen spectra and one electron ions (with major caveats) and does so, but, as discussed in subsequent chapters, fails at describing any atom or ion containing two or more electrons. 

The most concerning issues regarding the SQM model of the hydrogen atom are the required breaks with classical physics.  Accepting that SQM, as done herein, is able to predict the Rydberg series for hydrogen, does not make it immune to criticism on the basis of the non-physical nature of the electron required.  Indeed, the electron shape is never discussed, rather only the shape of the wave function, from which a probability distribution is computed. 

As will be argued in the Chapter 4 of this monograph, the wave function, which purportedly occupies all space, is only consistent with one shape for the actual electron (Figure 3-6). 

That is, (and this is clearly required by the mathematics of quantum physics the bound electrons in an atom in SQM), electrons are infinitely small particles for which the position and energy are “uncertain” and only described by a probability distribution, derived from a so-called wave function solution to Schrodinger’s equation.

Figure 3-6: SQM Probability Distributions.

The above are pictorial representations of the statistically most likely locations for electrons, which are zero-dimension point charges, in hydrogen ground state (s) and some excited states (p,d,f,g).  

These figures are not intended to be illustrations of bubbles or surfaces.  The shapes (red and blue) are probability distributions in 3 dimensions, with high probability of finding the electron throughout the inside of each lobe in the drawings, and some probability of finding the electron everywhere in the universe. For ordinary ground state hydrogen, the “s” probability prevails, indicating the electron in the ground state is very likely in the nucleus, a very unphysical solution as that implies a very low energy state.  How does the electron, once near the nucleus, gain the energy required to place itself at a greater distance from the nucleus?

Conclusion: SQM is nuts.   

It is also notable that all other atomic species in SQM are assumed to have the same set of probability distributions, even though for these multi-electron systems the spectrometer determined energy levels are never even close to that of the Rydberg formula.  This lack of fit is not surprising as multi-electron systems have forces not found in one electron systems such as electron-electron interaction, magnetic fields arising from other electrons, and partial screening of nuclear forces.  Thus, employing the one-electron model for multi-electron systems is risible.

SQM Interpretations

The SQM wave function has no obvious physical meaning, after all, it is just a probability distribution. It is thus generally “interpreted.” 

In this essay, the most widely accepted interpretation (Copenhagen) of the wave equation will be assumed: elementary particles such as electrons are infinitely small particles that have a probability of existing somewhere, as described by solutions to the Schrödinger wave equation. 

Other interpretations include that of Bohm: electrons are infinitely small particles, the behavior of which is described using classical physics, that “ride a wave,” however, this model is philosophy/metaphysics.³  It is not a quantitative mathematical model.   Its own definition requires more than the Schrodinger equation, specifically some as yet undetermined physics, or hidden variables.

Beyond the Copenhagen and Bohm interpretation are these:

• Many worlds.

• Spontaneous-collapse.

• Informational.

• Relational.

• Transactional.

All employ the same mathematics but cannot agree on a form of physical reality that underlies the math. 

It is also notable that according to SQM, in distinct contrast to the GUTCP, at a scale greater than h-bar, classical physics equations again describe all behavior. That is, according to SQM there are two realms of physical laws.  At very small scale, order of atomic scale, the non-classical Schrodinger model applies, but at larger scales classical physics pertains.   The two models are proclaimed to match at a scale around the scale of h-bar.  This agreement is known as the Correspondence Principle, as in: at a scale of around h-bar the classical and SQM models provide the same descriptions of matter. 

Really?

The GUTCP Hydrogen Atom

The GUTCP math for the hydrogen atom also produces the measured energy levels of hydrogen. The basic math is a force balance, the same force balance used in orbital mechanics and described in more detail in the next chapter: 

In the next chapter it will be demonstrated that the math is simple algebra, readily solved.  It will be shown to be far simpler math than a wave equation, and unlike the wave equation, requires no approximations. There is also no “parameter optimization” for multi-electron systems.  There are no variable parameters.

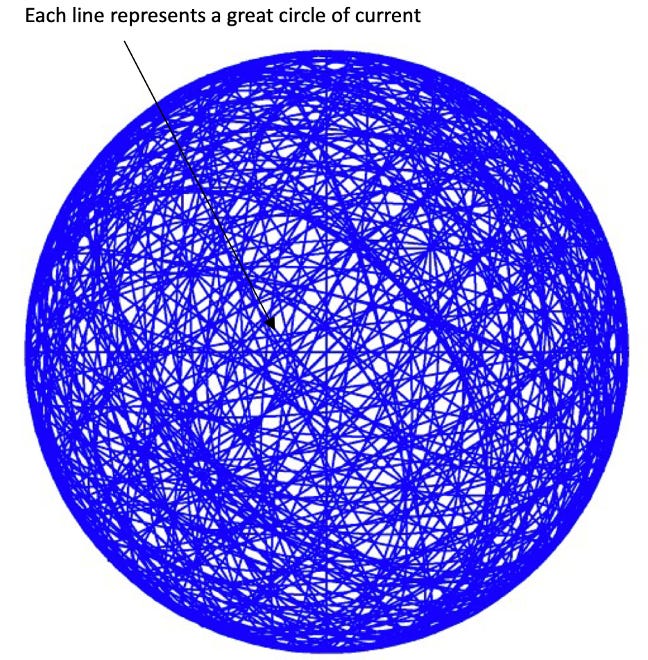
And this simple math leads to a very precise model for the bound electron: a bubble of charge of a specific radius upon which there is a pattern of great circle currents (Figure 3-6).⁴  There is no probability.  The electron has a precise shape. In every state, ground state and excited states, the electron is the same bubble shape, only with a different radius, and the current loops move at different velocities. 

Figure 3-6: The GUTCP Electron. All electrons in atoms and ions are spherical bubbles composed of great circles of current.  In a multi-electron system there are multiple orbitspheres of different radii set one inside the other like a set of Russian dolls. The speed of the current, and diameter of each orbitsphere is solved using classical physics equations. There is no probability, rather the electron is a precisely shaped physical object. There is no “uncertainty.”  There is no Correspondence Principle.  And the magnetic behavior arises, as per classical physics equations, from the motion of the current loops. 

Given that one cannot pick a winner from the two theories, the GUTCP and SQM, at the level of ordinary hydrogen and other one electron systems (e.g. He+) based on spectral fit, neither is debunked by the hydrogen data.

Scientifically speaking, if two theories can accurately describe the same data, neither is debunked.  To distinguish the preferred model, other experiments are required.  In the case of SQM vs. the GUTCP, the models are next compared for multi-electron systems.   For example, the outcomes of the two approaches are compared extensively for helium in the next chapter of this monograph. 

Spoiler alert: the GUTCP scores a huge win, and SQM is debunked.  

As noted, neither model is debunked at the hydrogen level, but there are some intuitive advantages to GUTCP.  SQM models the electron as a statistical “thing” in which an infinitely small particle has a probability of being somewhere, with some energy and momentum uncertainty, as defined by a wave function. Whatever that means.

The non-physical nature leads to the multitude of physical interpretations, Copenhagen, many worlds, etc, as discussed previously.

Contrast the squishy, ephemeral, 3 N dimensional (N is number of electrons in the system), probabilistic, rejected classical physics, non-trajectory, etc. nature of bound electrons in the SQM model, with the always 3-dimensional, absolute physical shape, classical physics equations only, basis of the GUTCP model.  The electron model of GUTCP  easily understood and intuitive. 

The electron of SQM? Not so intuitive.    

According to the GUTCP, elementary particles are three-dimensional objects with properties predictable using classical physics. In particular, all electrons bound to atoms are described as thin (“gravitational thickness”) spherical physical bubbles, orbitspheres, symmetrically surrounding the nucleus, with great circle surface currents that collectively produce, again based on classical physics, the measured angular momentum and magnetic moments of atomic bound electrons.

If the ion or atom has more than one electron, then each is an orbitsphere!  They are nested, the largest and least strongly bound, at the outside, the smallest and most tightly bound, nearest the nucleus. They do not touch in the model employed by this author, a slight modification from the original postulated by R. Mills.

In multiple electron systems, the electrons, all of the same spherical shape, are nested one inside the other like a set of Russian dolls.

Tests of the GUTCP model against accepted data, and predicted experimental outcomes, are the bulk of the following chapters.  It will be shown that GUTCP passes all tests!

The GUTCP:  Simple math, and simple reality. 

Summary

To re-phrase/repeat: the GUTCP is a fundamentally new/old approach that is completely inconsistent with SQM.  The GUTCP assumes elementary particles are 3-dimensional physical objects the behavior of which can be predicted entirely by classical physics, meaning it employs no equations posited post-1872.  In GUTCP, there is no Schrodinger equation.  GUTCP proponents argue and can demonstrate that it precisely predicts many phenomena, including the spectra of all atoms and free ions and the observed magnetic behavior.

It also predicts the existence of Hydrinos, which are predicted to be hydrogen atoms in which the electron is closer to the nucleus than the Bohr radius, a state which can’t exist according to SQM. 

The match of hydrogen models to measured parameters is nearly a tie for GUTCP and SQM, although only the former is physical and intuitive, and the latter is not.  In the following chapter the precision of the two models for predicting the observed spectra of atoms and ions larger than hydrogen is compared.

It is a huge win for GUTCP and suggests SQM for multi-electron systems is nothing but mathematical gibberish, or possibly simply a model that is professionally expedient for physicists to accept, or both.

Chapter 3 Personal Notes

Note to the reader: each chapter will include my personal experience with Dr. Mills, the GUTCP, and Hydrino science.

I. Nearly three decades ago, while quaffing beer and eating calamari late at night in a Princeton, NJ bar, Randy told me that one of his inspirations for a spherical orbitsphere was that electrons must have a geometry that does not produce a dipole, as electric dipoles are never found for atomic species, even in strong fields.  He postulated that any particle model of bound electrons would fail due to this observed absent dipole property.   He reasoned that only a spherical shape enclosing the nucleus would naturally fail to form a dipole. 

II. A PhD is a valuable asset, particularly as an entry card to high professional status.  The process to obtain one, however, can be a trap. Trap? To obtain a PhD in science or engineering requires devotion, day and night for five or more years, to a narrow sub-set of a narrow field. It is sometimes said:

A PhD signifies you know more and more about less and less, until you know everything about nothing. 

A physicist who spends five or more years mastering the use of advanced optical telescopes is likely to remain, for the entirety of his career, locked to problems associated with advanced optical telescopes.  The expertise gained by five tough years, working at sub-minimum wage pay, leads to recognition as an “expert,” and that is the capital/intellectual capital that will fund a career. Why throw it away?

Post-PhD, the academic profession will continue to demand demonstrations of expertise of those who hope to climb the ranks:

• Postdoc.

• Assistant professor.

• Tenure.

• Associate professor.

• Professor.

• Department chair.

• Dean.

This progression requires our exemplary optical-telescope-astrophysicist to produce publishable observations from data collected by the best space telescopes, gain invitations to international meetings where he will interact with others in the field working with optical telescopes, who he hopefully will impress.

Perhaps the most defining requirement will be the need to obtain grants and pass all tenure hurdles.  Scientific heretics are rarely invited to professional meetings, and extremely likely to have their papers rejected at “best” journals.  Government grants are unlikely for those who posit heresy as well.

Hence, tenure is rarely achieved by those who upset or question the established and powerful in the field. 

It is not too much of an exaggeration to say Rule #1 for getting tenure: do not question authority.  Example: the Dean of Engineering at Penn State indicated this was the basic reason my promotion to full professor was delayed. 

To paraphrase his comment on the delay: “It would enhance your chances of promotion if your work was not to stray from the accepted models in your field.  There is no advantage for you to antagonize senior members of the community.” In other words, publication rate, teaching, service, grantsmanship, were all better than fine, but…

Also, tenure is certainly less likely to be granted to an optical telescope astrophysicist who wakes up one day and identifies as an entomologist specializing in leaf cutter ant colonies in Central America.  Stick to your narrow field…or else.

It could be argued that once a reasonable position is obtained, for example tenure and associate professor status, our optical telescope astrophysicist may cautiously, probably obliquely, criticize some established paradigms in the field.  Perhaps our young scientist is getting intellectually restless and wants to begin working in some other fields.  These “rebellions” happen, but only in a small fraction of cases.

Why?  First consider the optimal timeline: four years of college, five years of graduate school, one year of post-doc, seven years until promotion.

Our young scientist, just tenured, is not really that young any longer at around age 35.  After so many years of somewhat obsequious professional behavior, leading to success, “behaving” becomes a habit that is hard to break.  

Indeed, our fellow has invested a lot of effort in developing contacts in senior positions in academia, editors at the proper journals, and professionals at the government agencies making grants in the field. Perhaps our fellow has a family.  Fighting the standard paradigm risks a great deal, and the return for the risk is typically minimal. 

Consider: your average faculty member will accede to absurd requirements to avoid trouble.  They/them.  Pledge to DEI. Whatever.

The above is a partial answer to the question that has so often been posed to me: why are there not more physicists and other scientists working to either critique or improve the GUTCP?  Why are there so few efforts to conduct experiments designed to debunk it? 

In brief, such efforts are not likely to lead to promotion, accolades from the community, additional grants, more students, invitations to meetings, editorships, etc.

Apropos of the above model of the standard behavior to anticipate from scientific leaders are my interactions with a number of senior scientists over the years.

While working at Los Alamos National Lab for one decade of my life, I had, through natural professional processes, interactions with many well-respected, senior, technical leaders.

One individual led a group that focused on plasmas, an expertise I shared, so we had reasons to collaborate.  He was one of a very few at the lab who would even engage me on the issue of the GUTCP.  Most ran, sometimes literally, away! We had intermittent discussions about the GUTCP, taking place over more than a year.  The discussions were open and professional.  My interlocutor was clearly sympathetic to the case for a new quantum model, however; when pressed to take part in some experimental studies designed to test the model he demurred:

“What’s in it for me?”

That is a direct quote, not a paraphrase. I interpreted that to mean: “why should I risk my excellent reputation by getting involved with something controversial?”

Another example from the same time period: I worked with a senior, well-known, engineering professor at the University of New Mexico.  He was my titular “boss” while at the university in my role as Distinguished National Lab Professor at UNM, and our families socialized together occasionally.  He became interested in the GUTCP and agreed to work, with financial support from Brilliant Light Power, on verifying the mathematics of the helium atom employed in the GUTCP model.  The final result: he verified the computations.  Does his name appear anywhere in the GUTCP literature? No. He was not interested in public involvement in anything so controversial.  The career risks, which I believe he felt were like a tsunami of negativity, were simply too great. 

My personal experience?  My embrace of the GUTCP was not without consequence.  For example my interactions with the two scientists noted above took a turn for the worse after our GUTCP interactions “failed,” and I attribute that to my embrace of the unorthodox.

Yes, there are consequences to iconoclast behavior. The risks are real but exaggerated.  Risk takers will take some hits in social and professional standing but survive and may even thrive.  In my case, the gain in psychic income and sense of enhanced freedom, from involvement in and contribution to, something extraordinary outweighed the limited loss in stature.

And yes, I was promoted to full professor, albeit a bit late.  

Take the above template of the politics of science and project it onto the general experience of BLP with the science community.  Indeed, consistent with the above remarks, most mainstream journals automatically reject BLP work.  Generally, neither an editor nor a reviewer finds it “career enhancing” to publish paradigm shifting concepts. 

Folks weigh consequences before seriously considering the science.  The results of publishing BLP work might not be limited to losing an editorship or position on the editorial board, it might result in loss of grant funding!  No one wants to take that risk!   

For the same reasons,  it was difficult for BLP to obtain the opportunity to make presentations at international meetings. Still, the work is published, generally in second tier journals, or new journals.  Or perhaps in journals outside the reach of the US community? As the saying goes, one cannot be a prophet in his own land…

The “community” is always willing to let paradigm shifts die on the vine. Perhaps the correct word is shunning?

1. C.H. Chou, and J. Phillips, “Platinum metal etching in a microwave oxygen plasma,” Journal of Applied Physics 68(5), 2415–2423 (1990).

2. C.-K. Chen, T.-C. Wei, L.R. Collins, and J. Phillips, “Modelling the discharge region of a microwave generated hydrogen plasma,” J. Phys. D: Appl. Phys. 32(6), 688–698 (1999).

3. D. Bohm, and B.J. Hiley, The Undivided Universe: An Ontological Interpretation of Quantum Theory (Routledge, London; New York, 1993).

4. What is current?  A word with many meanings, but as employed in the description of the orbitsphere, it is the steady motion of charged species in a loop, not the collection of the material of the current somewhere. For example, it is not equivalent to a current of water in a river adding water to a basin to create a lake.  Current in an orbitsphere, or in an electric circuit (Kirchoff’s Law), does not lead to a build-up of charge anywhere, not on the orbitsphere and not in a place on the circuit.  The charged material  (e.g. the great circle negatively charged loops of the orbitsphere) moves in a complete cycle.  At any point in time the charged material has the same concentration at every point in the loop, and is moving with the same velocity.   A good analogy is with the movement of water. A simple, ideal, water circuit: i) Heat causes ocean water to evaporate, ii) The evaporated water rises in the atmosphere until it cools and forms clouds.  iii) The clouds are driven by wind over land.  iv) Rain falls on the land. v) The water from the rain enters rivers, and eventually returns to the ocean. Repeat the cycle. There is a constant “current” of water, but it does not build up anywhere at least not in the ideal case. The current loops of the orbitsphere are made of a constant density of negatively charged material that is in moving at a constant velocity in the frame of the electron.  Another analogy: a loop of current on an orbitspere is like a hoola hoop with the same charge density on its surface at all points, actively spinning at a constant rate.  The reader is invited to create his own analogies...